Puzzle 1
Below you will find well-known eight-letter words, with only their endings remaining.
Can you find the words?
-----rue
-----jar
-----dil
-----any
-----pox
-----gth
-----elf
-----oia
-----ige
-----hog
-----too
-----dix
-----fin
-----upt
Puzzle Copyright © Kevin Stone
Share link: https://www.brainbashers.com/
Puzzle 2
Place the words below into pairs.
You will then have 5 lots of eight letters, each of which is an anagram of at least one other word.
Can you find the 5 pairings, and at least one anagram each?
peer
time
date
wall
june
tone
snob
seat
pale
veil
Puzzle Copyright © Kevin Stone
Share link: https://www.brainbashers.com/

Puzzle 3
Hidden in the grid below are six hidden animals.
The letters are in sequence, but you move from letter to letter using the moves of a chess knight.
Once you have crossed off all of the animals, you will be left with seven unused letters.
What animal do these letters spell?

The moves of a knight are:
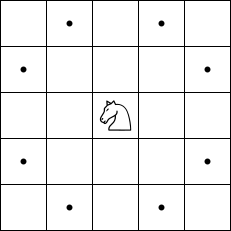
Note: this puzzle is not interactive, and the letters cannot be clicked.
Puzzle Copyright © Kevin Stone
Share link: https://www.brainbashers.com/
Puzzle 4
Below, you will find 10 seven-letter words, with only their endings remaining.
Can you find the words?
----igy
----aoh
----erg
----ynx
----lth
----mox
----ovy
----esh
----awe
----ipt
Puzzle Copyright © Kevin Stone
Share link: https://www.brainbashers.com/
Note: BrainBashers has a Dark Mode option. For BrainBashers, I'd recommend not using your browser's built-in dark mode, or any dark mode extensions (sometimes you can add an exception for a specific website).
