Puzzle 1
Last week I travelled from London to Leeds, a distance I measured as 174 miles.
I started at 9.15am and completed the journey with an average speed of 40 miles per hour.
On the way back, in the evening, I travelled exactly the same route, starting at 5.15pm. The traffic was light, and I completed the journey with an average speed of 60 miles per hour.
What was the overall average speed for the round trip?
The answer is not 50 mph.
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: https://www.brainbashers.com/puzzle/ztah
Hint
Remember that Time = Distance ÷ Speed, and Speed = Distance ÷ Time.
Answer
48 miles per hour.
Reasoning
Using:
Time = Distance ÷ Speed
Speed = Distance ÷ Time
The outward journey took 174 miles ÷ 40 mph = 4.35 hours.
The return journey took 174 miles ÷ 60 mph = 2.9 hours.
So I travelled a total of 2 x 174 = 348 miles. I did this in a total of 4.35 + 2.9 = 7.25 hours.
My overall average speed was therefore 348 miles ÷ 7.25 hours = 48 miles per hour.
Puzzle 2
Can you find a five-digit number that has no zeros, and no repeated digits, where:
The first digit is a prime number.
The second digit is the fifth digit minus the first digit.
The third digit is twice the first digit.
The fourth digit is the third digit plus three.
The fifth digit is the difference between the first digit and the fourth digit.
Don't forget that 1 isn't prime, the prime numbers start with 2, 3, 5, …
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: https://www.brainbashers.com/puzzle/zcvz
Hint
Start with the possible answers where the first digit is a prime number, and then look at the third digit.
Answer
23,475.
Reasoning
By (1), the first digit is prime:
2----
3----
5----
7----
By (3), the third digit is twice the first digit, so we can eliminate 5---- and 7----:
2-4--
3-6--
By (4), the fourth digit is the third digit plus three:
2-47-
3-69-
By (5) the fifth digit is the difference between the first digit and the fourth digit:
2-475
3-696
We know from the introduction that no digit is repeated, so we can eliminate 3-696. And, by (2) the second digit is the fifth digit minus the first digit:
23475
Puzzle 3
My local bus company has recently expanded and no longer has enough room inside its garage for all of its buses.
Twelve of their buses have to be stored outside.
If they decide to increase their garage space by 40% they will have enough room for all of their current buses, plus enough room to store another twelve in the future.
How many buses does the company currently own?
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: https://www.brainbashers.com/puzzle/zwol
Hint
Think about the 40%, and how many buses that represents.
Answer
72 buses.
Reasoning
The garage is currently full, with 12 more buses outside.
If we increase the garage space by 40%, there will be enough space to house those 12 buses, plus 12 more in the future – a total of 24 buses.
This means that 40% of the current garage capacity is 24 buses.
Therefore, 10% of the current garage capacity is 6 buses.
Therefore, 100% of the current garage capacity is 60 buses.
So, the current garage holds 60 buses, and there are 12 more outside.
Therefore, we have a total of 72 buses.
Double-Checking
They currently have 72 buses, 12 of which are outside, so 60 are inside.
Increasing the garage space of 60 buses by 40% will give room for 60 x 1.4 = 84 buses.
Which is the 72 they currently own, with space for 12 more buses in the future.
This confirms the answer.
Puzzle 4
Within the BrainBashers school, the science department has three disciplines.
In total, 280 students study chemistry, 254 students study physics, and 280 students study biology.
In total, 97 students study chemistry and physics, 138 students study physics and biology, and 152 students study chemistry and biology.
73 students study all three disciplines.
Can you determine how many students there are in the science department? The answer is not 814.
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: https://www.brainbashers.com/puzzle/zzyu
Hint
How many students study chemistry and physics, but not biology?
Answer
500 students.
Reasoning
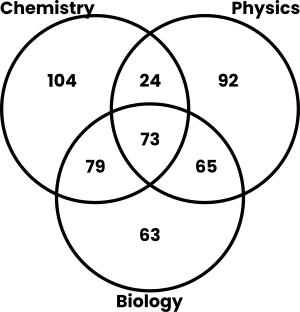
The answer is more easily seen if three intersecting circles are drawn, and the numbers inside each section are worked out.
We know that 73 students study all three disciplines, which allows us to work out the numbers.
97 students study chemistry and physics, so 97 – 73 = 24 study just chemistry and physics.
138 students study physics and biology, so 138 – 73 = 65 study just physics and biology.
152 students study chemistry and biology, so 152 – 73 = 79 study just chemistry and biology.
280 students study chemistry, so 280 – 73 – 24 – 79 = 104 study just chemistry.
254 students study physics, so 254 – 73 – 24 – 65 = 92 study just physics.
280 students study biology, 280 – 73 – 65 – 79 = 63 study just biology.
For a total of: 104 + 92 + 63 + 24 + 65 + 79 + 73 = 500 students.
next >
Note: BrainBashers has a Dark Mode option. For BrainBashers, I'd recommend not using your browser's built-in dark mode, or any dark mode extensions (sometimes you can add an exception for a specific website).
