Puzzle 9
Yesterday I went for a short bicycle ride around the local lakes. As the weather was very hot, I rode in shorter stages than I'd normally ride.
In Stage One, I rode half of the overall distance.
Stage Two saw half of the remaining distance plus 35 metres covered.
Stage Three covered three-quarters of the remaining distance.
Stage Four completed half of the remaining distance, plus 75 metres.
Stage Five completed the journey with a final burst of 150 metres.
How far did I cycle in total?
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: https://www.brainbashers.com/puzzle/zofe
Hint
Try working backwards.
Answer
7,340 metres.
Reasoning
Stage 5's clue tells us that 150m was left. So the distance left at the start of Stage 4 must have been:
Dist4 = Dist4 + 75 + 150
—————
2
Which simplifies to give Dist4 = 450m.
Stage 3's clue tells us that 450m was one quarter of the remaining distance, so Dist3 = 1,800m.
Stage 2's clue tells us that the distance at the start of Stage 2 was:
Dist2 = Dist2 + 35 + 1800
—————
2
Which simplifies to give Dist2 = 3,670m.
Stage 1's clue tells us that 3,670m was half the overall distance, which means the entire ride was 7,340m.
Double-Checking
Starting with 7,340m:
Stage 1: cycled 3,670m, leaving 3,670m
Stage 2: cycled 1,870m, leaving 1,800m
Stage 3: cycled 1,350m, leaving 450m
Stage 4: cycled 300m, leaving 150m
Stage 5: cycled 150m, leaving 0m
Puzzle 10
At midnight at the start of January 1st, Professor Stone set two old-fashioned clocks to the correct time.
One clock gains one minute every hour, and the other clock loses two minutes every hour.
When will the clocks next show the same time as each other?
When will the clocks both show the correct time?
Puzzle Copyright © Kevin Stone
workings
hint
answers
print
Share link: https://www.brainbashers.com/puzzle/zdjb
Hint
In order for the clocks to show the same time (e.g. 2 o'clock), what must the total time gained by one, and lost by the other, total?
Answers
Midnight, 10 days later, when they will both show 4 o'clock.
Midnight, 30 days later, when they will both show 12 o'clock.
Reasoning #1
In order for the clocks to show the same time, the total time gained by one, and lost by the other, must be 12 hours.
For example, if the first clock were to show 2 o'clock, it would have gained 2 hours. In order for the second clock to also show 2 o'clock, it would have had to have lost 10 hours. This is a total of 12 hours gained and lost.
We know that for every hour that passes, the first clock gains one minute, and the second clock loses 2 minutes, for a total time gained and lost of 1 + 2 = 3 minutes.
The total time gained and lost will equal 12 hours when 12 x 60 ÷ 3 = 240 hours have passed. 240 hours = 10 days.
The first clock will have gained 240 x 1 minutes = 240 minutes = 4 hours.
The second clock will have lost 240 x 2 minutes = 480 minutes = 8 hours.
So, they will both show 4 o'clock, 10 days later.
Reasoning #2
In the first answer, we can see that 10 days later, the clocks both show 4 o'clock.
If we move forward another 10 days, both clocks would show 8 o'clock.
If we move forward another 10 days, both clocks would show 12 o'clock.
This will now be the correct time.
So, they will both show 12 o'clock, 30 days later.
Puzzle 11
Alex and Billie were rowing their canoe along the River Trent.
In the morning, they managed to row upstream at an average speed of 2 miles per hour.
They then stopped for a spot of lunch and a nice rest.
In the afternoon, the pace was a little easier as they were now rowing downstream back to their starting point, and managed an average speed of 4 miles an hour.
The morning trip took them 3 hours longer than the afternoon.
How far did they row upstream?
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: https://www.brainbashers.com/puzzle/zoty
Hint
You can either fix the distance rowed and see what numbers work, or you can fix the number of hours.
Answer
12 miles.
In the morning, rowing at 2 miles per hour, they rowed for 6 hours. In the afternoon, rowing at 4 miles per hour, they rowed for 3 hours.
There are a number of ways of working this out, and here are two of them:
Method 1
If we assume the distance the rowed upstream to be D miles, we know the morning took (D ÷ 2) hours, and the afternoon took (D ÷ 4) hours, with a difference of 3 hours. So:
D D
- – - = 3
2 4
Multiplying throughout by 4 gives:
2D – D = 12
So:
D = 12 miles
They rowed 12 miles upstream.
Method 2
If we assume they rowed for H hours upstream, we know they travelled H x 2 miles in the morning. In the afternoon, they rowed for (H – 3) hours, and travelled (H – 3) x 4 miles. We know these distances are the same, so:
2H = (H – 3) x 4
Giving:
2H = 4H – 12
Rearranging gives:
12 = 2H
So:
H = 6 hours
They rowed for 6 hours upstream at 2 miles per hour, which is a total of 12 miles.
Puzzle 12
Starting in the bottom left corner and moving either up or right, adding up the numbers along the way, what is the largest sum which can be made?
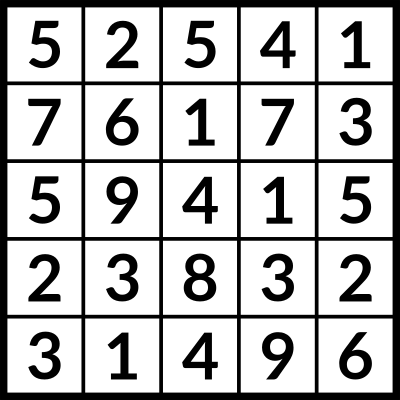
Note: this puzzle is not interactive, and the numbers cannot be clicked.
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: https://www.brainbashers.com/puzzle/zwsc
Answer
38.
< previous next >
Note: BrainBashers has a Dark Mode option. For BrainBashers, I'd recommend not using your browser's built-in dark mode, or any dark mode extensions (sometimes you can add an exception for a specific website).


