Puzzle 13
Start in the bottom left corner and move either up or right, one square at a time, adding up the numbers. What is the largest total you can make?
| 1 |
4 |
2 |
2 |
1 |
2 |
| 4 |
3 |
1 |
3 |
4 |
3 |
| 2 |
1 |
4 |
2 |
1 |
2 |
| 1 |
2 |
2 |
3 |
3 |
1 |
| 4 |
1 |
3 |
1 |
2 |
1 |
| 3 |
1 |
4 |
3 |
4 |
2 |
| 2 |
1 |
1 |
1 |
1 |
3 |
| 3 |
4 |
2 |
3 |
2 |
2 |
Note: this puzzle is not interactive, and the squares cannot be clicked.
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: www.brainbashers.com/puzzle/zngr
Hint
The answer is over 35.
Answer
The largest total that can be made is 37.
|
|
|
|
|
2 |
|
|
|
3 |
4 |
3 |
|
|
4 |
2 |
|
|
|
|
2 |
|
|
|
|
|
3 |
|
|
|
|
|
4 |
|
|
|
|
|
1 |
|
|
|
| 3 |
4 |
2 |
|
|
|
Puzzle 14
At the local sweet shop, three particularly nice sweets are on special offer.
A Nobbler is over three times the price of a Sparkle.
Six Sparkles are worth more than a Wibbler.
A Nobbler, plus two Sparkles costs less than a Wibbler.
A Sparkle, a Wibbler and a Nobbler together cost 40p.
Can you determine the price of each type of sweet?
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: www.brainbashers.com/puzzle/zqvp
Hint
Which sweet is the least expensive?
Answer
Sparkle = 4p
Wibbler = 23p
Nobbler = 13p
Reasoning
By (3) a Nobbler, plus two Sparkles costs less than a Wibbler, therefore a Wibbler must be the most expensive sweet.
By (1) a Nobbler is over three times the price of a Sparkle, therefore a Sparkle must be the cheapest sweet.
So the order of sweets, from the least to most expensive, is Sparkle, Nobbler, Wibbler.
If a Sparkle was 1p, by (2) a Wibbler could only be up to 5p, by (4) a Nobbler would cost at least 34p, which is more than a Wibbler and isn't allowed as the Wibbler is the most expensive sweet.
If a Sparkle was 2p, by (2) a Wibbler could only be up to 11p, by (4) a Nobbler would cost at least 27p, which is more than a Wibbler and isn't allowed as the Wibbler is the most expensive sweet.
If a Sparkle was 3p, by (2) a Wibbler could only be up to 17p, by (4) a Nobbler would cost at least 20p, which is more than a Wibbler and isn't allowed as the Wibbler is the most expensive sweet.
So a Sparkle must be at least 4p.
If a Sparkle was 4p, by (1) a Nobbler must be at least 13p, by (4) a Wibbler would cost 23p – this combination matches all of the clues and is a possible solution.
If a Sparkle was 4p and a Nobbler 14p, by (4) a Wibbler would cost 22p. This would not satisfy (3). And if we increase the price of a Nobbler, (3) is never satisfied.
If a Sparkle was 5p, by (1) a Nobbler must be at least 16p, by (4) making a Wibbler at most 19p. This would not satisfy (3).
If we increase the price of a Sparkle or Nobbler further, (3) is will never be satisfied.
Therefore, the only solution we came across must be the correct one.
Puzzle 15
A coin collector decides to divide his coin collection between his children.
The eldest gets 1/2 of the collection, the next gets 1/4 of the collection, the next gets 1/5 of the collection, and the youngest gets the remaining 49 coins.
How many coins are in the collection?
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: www.brainbashers.com/puzzle/zxnw
Hint
What do the fractions add up to?
Answer
There are 980 coins in the collection.
Reasoning
Using fractions:
1/2 + 1/4 + 1/5 = 19/20
The remaining 1/20 is 49 coins.
Therefore, the 20/20 must equal 20 lots of 49 = 980.
Alternative Reasoning
Using percentages:
50% + 25% + 20% = 95%
The remaining 5% is 49 coins.
If 5% is 49 coins, 10% is 98 coins, 100% is 980 coins.
Double-Checking
1/2 of 980 is 490
1/4 of 980 is 245
1/5 of 980 is 196
and the remaining 49 coins.
And 490 + 245 + 196 + 49 = 980.
Puzzle 16
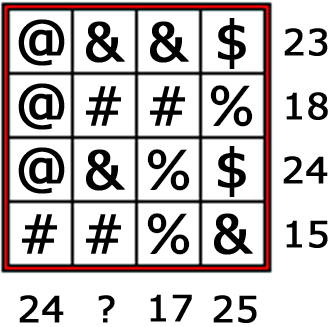
Can you find the sum of the second column?
Puzzle Copyright © Kevin Stone
workings
hint
answer
print
Share link: www.brainbashers.com/puzzle/znwn
Answer
14.
Reasoning
The values are: @ = 7, & = 4, $ = 8, # = 3, % = 5.
But...
...you don't have to find the value of every symbol!
The rows add up to 80, which means that the columns must also add up to 80.
24 + ? + 17 + 25 = 80.
? = 14.
< previous next >
Note: BrainBashers has a Dark Mode option – I recommend not using your browser's dark mode or extensions for BrainBashers

