Here we have a rectangular room, measuring 30 feet by 12 feet, and 12 feet high.
There is a spider in the middle of one of the end walls, 1 foot from the ceiling (A).
There is a fly in the middle of the opposite wall, 1 foot from the floor (B).
What is the shortest distance that the spider must crawl in order to reach the fly?
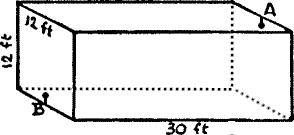
The Spider and the Fly – The Canterbury Puzzles, Henry Ernest Dudeney.
workings hint hide answer print
Share link – www.brainbashers.com
Answer
40 feet.
Explanation Diagram
If you imagine the room to be a cardboard box, you can 'unfold' the room in various ways, and each route gives a different answer.
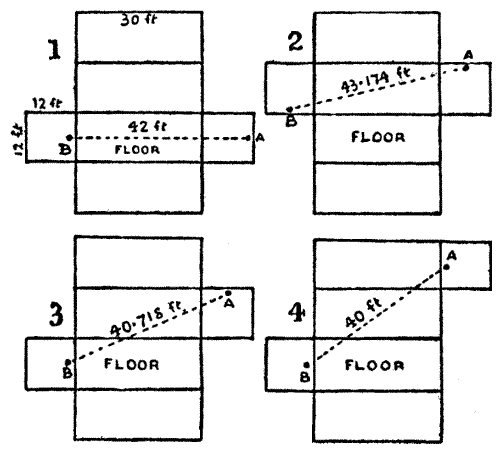
We can use Pythagoras' theorem (a2 + b2 = c2) to calculate the distances:
distance2 = horizontal2 + vertical2
distance = √(horizontal2 + vertical2)
Route #1
distance = 1 + 30 + 11 = 42 feet.
Route #2
horizontal = 6 + 30 + 6 = 42 feet.
vertical = 10 feet.
distance = √(422 + 102) ≈ 43.174 feet.
Route #3
horizontal = 1 + 30 + 6 = 37 feet.
vertical = 6 + 11 = 17 feet.
distance = √(372 + 172) ≈ 43.178 feet.
Route #4
horizontal = 1 + 30 + 1 = 32 feet.
vertical = 6 + 12 + 6 = 24 feet.
distance = √(322 + 242) = 40 feet.
Note: BrainBashers has a Dark Mode setting.